
The Keynesian Beauty Contest is a game theory experiment where a group of players are asked to guess a number between 0 and 100. The winner of the contest is the player(s) who guess closest to half the average of all the guesses.
This is an attempt to perform this experiment on Manifold. At close I will resolve the market to the option that is closest to half the rounded average of the probability weighted value of the options.
For instance if there are only two options, 25 with 25% chance and 50 with 75% chance I would resolve to (0.25*25+0.75*50)/2=21.88~=22. Since 25 is closest to 22, the market would resolve to 25.
I've added options for all the multiples of 10 to start with. If I've set this up right, you will be able to add your own answers. Keep these guidelines in mind when adding additional options (or your option will not be included in the experiment):
The option must be an integer between 0 and 100, inclusive.The option must be written in digit form (to avoid duplicates).
Should it not be possible to add options, please request the option you want added in the comments, and I'll add it.
As we have reached the limit of how many options can be added, no further options will be added. Every option except 92 and 93 is available.
Fine print
Percentages will be read visually from the market page.
Should invalid options be added, the resulting average will be divided by the sum of probabilities of valid options, to ensure consistent values.
For the resolution of this market "Other" will always be considered an invalid option. The only reason to bet yes on "Other" would be to speculate on options added in the future.
Each day starting Sep 9th I will add the option which would currently win, if it is not yet part of the options.
In case of discrepancies between the textual description and the spreadsheet calculation, the spreadsheet will decide.
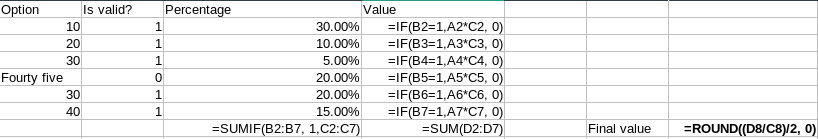
Here is a screenshot of a spreadsheet with formulas, showing how the final value will be calculated:
Similar markets
References
🏅 Top traders
| # | Name | Total profit |
|---|---|---|
| 1 | Ṁ4,204 | |
| 2 | Ṁ2,004 | |
| 3 | Ṁ1,587 | |
| 4 | Ṁ1,180 | |
| 5 | Ṁ1,094 |
I'm posting my calculation so that everyone has the opportunity to double-check my work, but my result matches the previously posted output of the script, so it should be fine. If there are no mistakes, expect a final resolution tomorrow morning (in ~8 hours for me).
https://drive.google.com/file/d/1XxLZ7njEWgRZuYD7GG7iSleFx5zblMbe/view?usp=drive_link
Thanks to playing! I'm excited to see how @DanMan314 's variant will go.
@DanMan314 In the last few minutes it took ~10 seconds for any bets to go through, so it was hard to quickly buy based on the current information.
And on top of that, if someone would have bought 11 to >30%, the mean would have dropped enough that it wouldn't be the correct answer anymore.
@FlorisvanDoorn Yea but presumably by the intermediate value theorem there's some value you can bid up to 30% or so such that you move the correct answer to it. Still really difficult to pull off, but I would have expected multiple people to try that kind of thing.
@DanMan314 Sure, but given that everyone else tries something else at the same time, it's a very risky strategy.
@DanMan314 feeling smug right now, 11 was just the upper end of the range that I was buying, precisely because it was so cheap.
@VitorBosshard Some values would go to 0.0%, but the weighted average of other values could still be be over 2.1
(lol I replied to the wrong comment thread)
@SavioMak Yes, specially because I also submitted 850 of 1 NO at the last minute. If that had gotten through after @Simon74fe 's order, it would have been gg for them and incredibly good for me.
@AndrewHebb My preliminary results land on 11 as well. I'm gonna post a screenshot of my spreadsheet soon to allow anyone to catch any errors (if there are any) and then resolve in ~12 hours.
@Joshua Imagine if Simon is just a big troll spending $40 to make everyone stare at this market for half an hour
@FlorisvanDoorn It would have pushed 1 above 90. The average for the other numbers would have been less than 0.1
@Simon74fe If the market rate for 1 would be 91% or higher, I think it would indeed resolve 1. That would have been expensive for me!