This market is a variation of my Keynesian Beauty Contest market (see https://manifold.markets/Toby96/keynesian-beauty-contest-5c4903348eb7)
As with the above market, the market will resolve based on the probability weighted average of all the options. Let's call this value v.
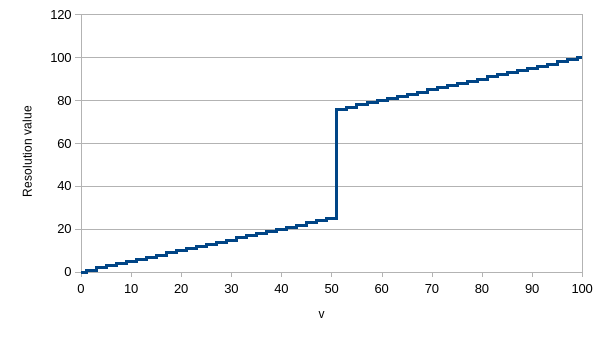
If v <= 50, the market resolves exactly as shown in the Keynesian Beauty contest market, that is to 0.5v rounded to the nearest integer.
If v > 50, the market resolves to v+(100-v)/2 rounded to the nearest integer.
Essentially this amounts to a Keynesian Beauty Contest in opposing directions.
Note that if the option does not exist, the option(s) that are closest will be chosen instead. In no case will the market be resolved to the "Other" option
Example calculation
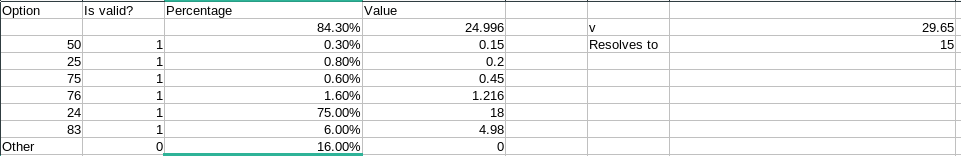
There's only two options, 30 with 25% and 70 with 75%. v=0.25*30+0.75*70=60
The second resolution criteria applies, meaning the market would resolve to the option closest to 60+(100-60)/2=80 (ie. 70).
On this market you will be able to add your own answer options. These options will only be considered valid if the following guidelines are followed (Otherwise they will be disregarded for the calculations and resolution of the market):
- The option must be an integer between 0 and 100, inclusive.
- The option must be written in digit form (ie. "45", not "fourty five").
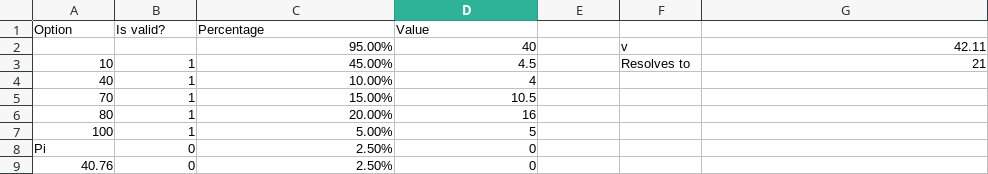
A screenshot illustrating how exactly this calculation will be performed is shown below:
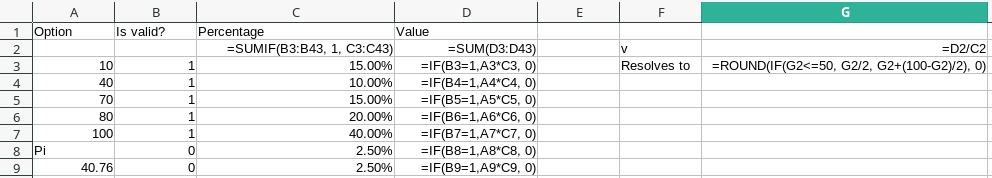
v>50:
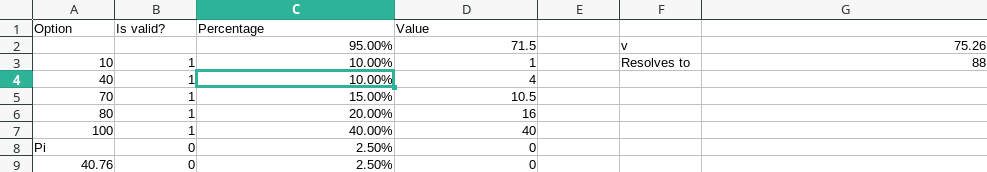
v<=50:
Fine print
Percentages will be read visually from the market page.
Should invalid options be added, the resulting average will be divided by the sum of probabilities of valid options, to ensure consistent values.
For the resolution of this market "Other" will always be considered an invalid option. The only reason to bet yes on "Other" would be to speculate on options added in the future.
In case of discrepancies between the spreadsheet and the description, the spreadsheet will be considered correct.
🏅 Top traders
| # | Name | Total profit |
|---|---|---|
| 1 | Ṁ967 | |
| 2 | Ṁ213 | |
| 3 | Ṁ175 | |
| 4 | Ṁ5 | |
| 5 | Ṁ0 |
@ChristopherRandles Absolutely, haha! Maybe you could do it next time you run this market, if you do so again?