Will a quantum computer factor a 6-bit number before 2026?

1kṀ3406

2026

88%

chance

ALL

Background: A 2019 paper showed the IBM Q System One was able to factor 15 and 21, but unable to factor 35 due to accumulating errors. Will a quantum computer factor a number greater than 31 by 2026?

Must use a non-classical algorithm like Quantum Fourier Transform/Shor's Algorithm to count, and without pre-compilation.

Quantum Computing

Get

1,000

to start trading!

People are also trading

Will a quantum computer perform a calculation by 2026 that is impossible for any classical supercomputer?

41% chance

Will a quantum computer prove capable of breaking 2048 bit RSA encryption by 2030?

21% chance

Will Quantum computing break RSA encryption before 2030?

34% chance

Will a quantum computer factor the RSA-2048 challenge number before 2035?

47% chance

Will a quantum computer factor the RSA-2048 challenge number before 2030?

8% chance

Will a quantum computer factor the RSA-2048 challenge number before 2075?

78% chance

Will a quantum computer factor the RSA-2048 challenge number before 2040?

65% chance

Will a quantum computer factor the RSA-2048 challenge number before 2050?

81% chance

will i get a quantum computer in my lifetime?

67% chance

Will a quantum computer factor the RSA-2048 challenge number before 2100?

Sort by:

@xyz what does 'without precompilation' mean here?

Does this count:
https://github.com/Curtisflo/QuantumFactorization

@QuantumObserver Also does it need to find all factors or just a factor?

@QuantumObserver I don't know enough about quantum computation to be honest, so I'm willing to take suggestions. The Github you linked says it uses it uses "classical post-processing" which I guess should be fine?

@xyz So here are screenshots of my runs of the code linked above for 77. It did use time on an IBM QPU (127 qubits) and produced correct factors. 1st screenshot uses an advantageous initial guess for the period finding (period = 2), but 2nd and 3rd show period = 3 and period = 10. Not as amazing, but still, able to find factors.

There are some philosophical issues here that Craig Gidney pointed out recently, once of which is that these numbers are so small that even an RNG output for the quantum parts of the algorithm could get you to the right answer. I don't see a good way to get around this without just voiding the question entirely.

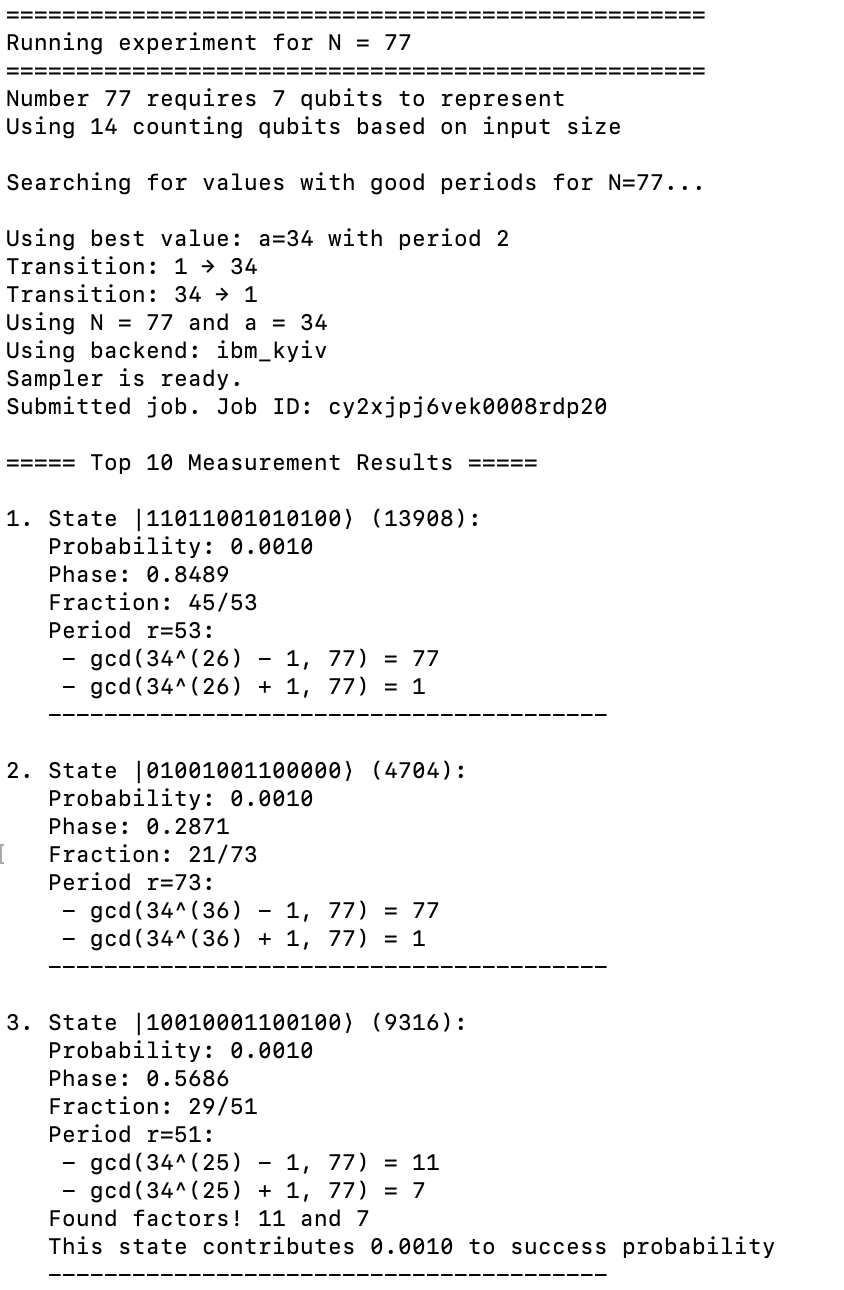
Turns out that larger semiprimes have been factored with adiabetic quantum computation and quantum annealing, so I’ve changed the question to refer exclusively to Shor’s, which was the original intention.