All irrational numbers can be written as continued fractions, like the one below.

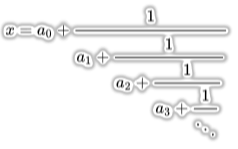
It turns out that, as N approaches infinity, the geometric mean of the first N a_i coefficients converges to a constant called Khinchin's constant for almost all (i.e., all but a Lebesgue null set of) numbers. This property can be called Khinchin;s property.

Although almost all numbers have this property, and some well-known constants are believed to have it, there are no natural examples of numbers that have been proven to have it. Specifically, no numbers that weren't explicitly contructed to provide an example have been proven to have Khinchin's property.

By 2040, will there be a proof that some number that was originally defined for a purpose other than providing an example of Khinchin's property has Khinchin's property? This would include a proof that Khinchin's constant itself has Khinchin's property.