
After inauguration day (last one was January 20, 2021), we compute the difference of the MSCI USA index (end of day) to one year before.
One answer resolves NA depending on the presidential election outcome.
The other answer resolves YES if the MSCI USA grew by more than 11%.
The other answer resolves NO if the MSCI USA grew by 11% or less.
Close date will be adjusted to the inauguration date.
The general idea: How good is each candidate for the US economy?
A stock price is determined by the company's future stream of earnings. So we use the MSCI USA index as kind of a prediction market for the US economy outlook. In contrast to GDP, this is a leading indicator and available. It does only reflect a part of the US economy though.
Stock prices anticipate the election outcome, so there is (probably) no sharp change after the election. Instead we use a one year period over the election to measure how the votes influence the economic outlook. Also, there is data readily available for annual index growth rates. For example, from MSCI itself:
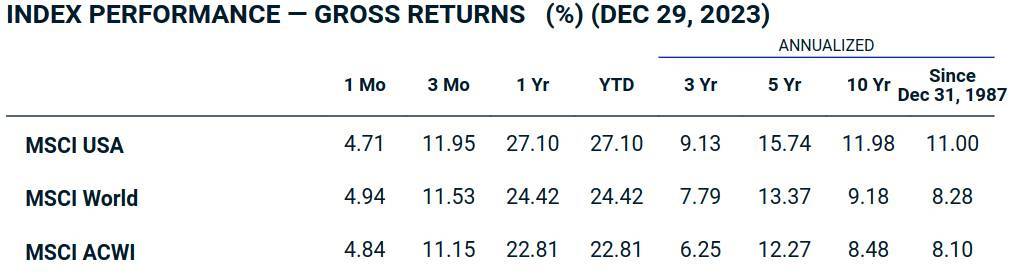
The 11% is simply the annual average since 1987. There is nothing relevant about this particular number other than it is a reasonable guess for a 50% base rate and a nice round number. The growth itself is not that interesting for voters, they care about the difference between the candidates. While we could also try to guess the growth rate, a distribution is harder to compare. Hence the simplified question ">11%?".
Inauguration day is a clear date where the election result is clear and stable. Even if the loser challenges the outcome, this should be resolved then.
Example resolution from last election
Last inauguration was January 20, 2021 and MSCI USA was at 3,937.121. One year before, on January 20, 2020 is was at 3,337.562. That is a difference of +599.559 or +18.0% and clearly >11%. Thus, a ">11% if Democrats win" would have resolved YES back then.
🏅 Top traders
| # | Name | Total profit |
|---|---|---|
| 1 | Ṁ24 | |
| 2 | Ṁ9 | |
| 3 | Ṁ9 | |
| 4 | Ṁ1 | |
| 5 | Ṁ0 |
Made a separate unconditional market to focus on the actual number: /marktwse/msci-usa-performance-in-2024
@marktwse This less precise variant is probably more helpful (at least until the election):
Note that the "10%" answer there could resolves YES or NO here.
Thank @SirCryptomind for hint at this.

I don't think this bias is really relevant here, but fascinating data nonetheless.